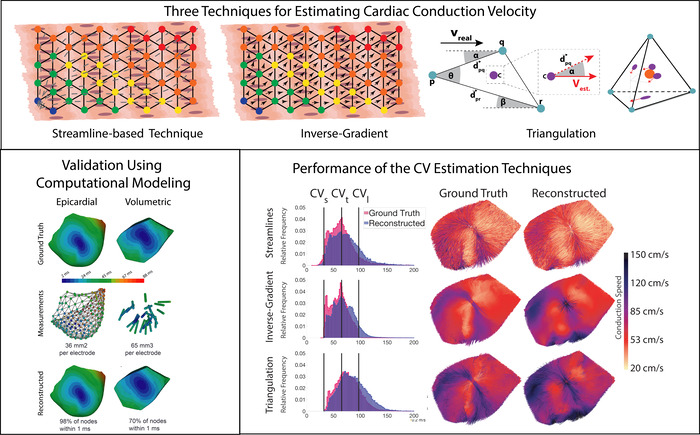
Cardiac conduction velocity (CV) is an important electrophysiological property that describes the speed and direction of electrical propagation through the heart. Accurate CV measurements provide a valuable quantitative description of electrical propagation that can help identify diseased tissue substrate and stratify patient risk. In this study, we explored a range of techniques for estimating epicardial and volumetric CV, and validated the performance of the techniques using whole heart image-based computational modeling. The CV estimation techniques implemented in this study (streamlines, triangulation, inverse-gradient) produce accurate, high-resolution CV fields that can be used to study propagation in the heart experimentally and clinically.
We were able to compare the performance of the novel streamline-based technique with that of two techniques from the literature (inverse-gradient and triangulation). The novel streamline-based technique can operate epicardially, and volumetrically, and was shown to be robust to interpolation-related artefacts. Furthermore, the streamline technique enhances visual interrogation of the CV fields due to the coherence and collinear vectors produced by the streamlines. We showed that RBF-based interpolation, using the sampling we achieved during our large animal experiments, are sufficient to adequately reconstruct epicardial and volumetric wavefronts with high-fidelity and perform accurate CV estimations in these domains. The inverse-gradient technique appears best suited for spatial analyses in the volume and epicardium when estimations of elementwise CV vectors are necessary, for example, with fibrosis overlap or during acute myocardial ischemia. Conversely, the streamline-based technique produces visually interrogative CV fields that emphasize propagation and may be particularly useful in the examination of reentrant phenomena and lines of block.

